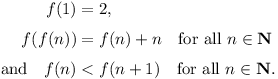34th INTERNATIONAL MATHEMATICAL OLYMPIAD
ISTANBUL, TURKEY
13th-24th JULY 1993
Report by ADAM McBRIDE (UK Team Leader)
In preparing this chronicle of events leading up to and during this
year’s International Mathematical Olympiad (IMO) I have been
influenced by the favourable response to last year’s report by Tony
Gardiner. Although Tony’s style is inimitable, I have tried to retain
his informal approach in what follows.
Mathematical Competitions
Before we proceed to the main business, a little evangelism is in order. In
UK schools we have many youngsters with great mathematical potential. Some
go on to realise their full potential but, alas, there are lots who do not.
At the age of 12 or 13, bright pupils are thirsting for knowledge and relish
a challenge. If these pupils are not stretched mathematically, their fertile
minds may turn to other things and be lost to Mathematics. Hard-pressed
teachers may not have time to generate “extension material” for their
abler pupils but can certainly make use of material generated by others.
It is here that mathematical competitions come in. There is now a range of
such competitions covering all years in secondary schools. Yet the existence
of such competitions is not well known to many teachers and even less well
known to pupils.
Most problems set in competitions require relatively little
mathematical knowledge and so can often be solved by using only
a little flair or insight. Yet they are far from “easy” and
teach pupils that not all problems can be solved in five
minutes flat. The patience and determination developed through tackling
problems are essential attributes for success in life in general. Since we
shall be talking mainly about Olympiads, let us remember the old ideal
that taking part is more important than winning. We can’t all be winners
but all can learn from the experience; many who struggle first time
round respond in the intended way by setting their sights that much
higher the next time.
In Australia 92% of high schools take part each year in the Australian
Mathematics Competition.
We have a long way to go before we can
match this figure but
my message to all those reading this document is
SPREAD THE WORD.
Those already involved should contact colleagues in neighbouring schools or
regions and whip up support. As a Scot, I am particularly keen to see
increased
participation from schools in Scotland, Wales and Northern Ireland. A small
amount of effort could produce considerable rewards. Go to it!
A number of competitions will be mentioned below, most of them falling
under the aegis of the British Mathematical Olympiad Committee (BMOC). For
further information on these and other competitions contact the BMOC
Secretary who is
Dr. Tony Gardiner, School of Mathematics and Statistics
University of Birmingham, BIRMINGHAM B15 2TT
After that clarion call, we can turn to the events leading up to the 1993 IMO.
Selecting the UK Team
The road to Istanbul was a long one. The first stage was the National
Mathematics Contest (NMC), run by the Mathematical Association and held in
November 1992. It attracted around 30000 entrants. This was a
substantial increase on the 1991 entry but we hope
the 1993 entry will be even larger.
The NMC paper contains 25 multiple choice
questions; the first 15 or so are accessible to most
contestants while the last few problems are more taxing.
Based largely on their performances in the
NMC, over 600 pupils entered
the British Mathematical Olympiad Round 1 (BMO 1), a
3½-hour paper with 5 questions held in January. Almost 90 pupils
were then selected to tackle BMO 2, another 3½-hour paper but with
just 4 questions and held in February. The selection for BMO 2 contained
a number of “wild cards” in the form of pupils whose BMO 1 marks may
have been disappointing but who nevertheless showed promise. Most of these
selections were duly vindicated and some of the younger contestants will have
derived great benefit from the experience.
We look forward to seeing what they achieve this coming year.
The BMO 2 field then had to be
reduced to exactly 20 for the residential Training Session at Easter.
This proved a tricky business, especially as we
always try to include 8 to 10 younger students.
The chosen 20 gathered at Trinity College, Cambridge for some systematic
instruction
during the period 15th-18th April. For three days, they had 4 or 5 intensive
2-hour sessions each day,
mostly spent on actually tackling problems rather than on formal
lectures, covering Algebra, Combinatorics, Geometry, Inequalities and Number
Theory. They also received advice on how to tackle hard problems and how to
write out solutions. Light relief was provided by a relay competition in
which a team of former UK IMO team members won narrowly, despite a spritely
performance from the Wrinklies (a distinguished subset of the instructors),
and some excellent work from the five student teams. After
surviving this mathematical onslaught, our young hopefuls were
rewarded with a 4½-hour examination called the Final Selection Test
(FST) on the last morning. This was intended to simulate a full-blooded
IMO paper and so contained just three questions.
The Training Session ended with the presentation of awards to all 20
students by Professor Sir Michael Atiyah, Master of Trinity College and
President of BMOC.
When the students had all left Cambridge, we were
left with the job of making the final selection, which again was far from
easy.
UK Team for 34th IMO
After the Training Session, the
UK team for the 1993 IMO was selected on the basis of all the information
then before us. The team was as follows:
Tom Fisher (Exeter College)
Alistair Flutter (Hills Road Sixth Form College, Cambridge)
Catriona MacLean (Harrogate Grammar School)
Alex Paseau (St. Paul’s School, London)
Luke Pebody (Rugby School)
Chuan Tze Teo (Dulwich College, London)
Reserve: Paul Hacking (Lancaster Royal Grammar School)
Team Leader: Adam McBride (University of Strathclyde,
Glasgow)
Deputy Leader: Christopher Bradley (Clifton College, Bristol)
Those in their last year at school who attended the Training Session but
who were not selected will have their chance at university to show what they
can do. The younger students who will be eligible for consideration again
next year are presently being kept ticking over by a correspondence course
kindly run by David Monk.
Final Preparations
During May and June the team embarked on a correspondence course with a
set of problems arriving every ten days. Of course, some already had plenty
of other examinations to prepare for but they survived. Our reserve,
Paul Hacking, deserves special mention for setting the pace throughout the
correspondence course; he would have been a worthy replacement
should any of the team have
suffered a mishap. From 3rd to 5th July, the team plus Christopher Bradley
and myself gathered for a weekend in Birmingham. The activities were
deliberately less structured than in Cambridge. For example, we all tried
some problems from current
issues of some journals including Crux Mathematicorum and the American
Mathematical Monthly.
We wrote up solutions to a couple
of problems together and sent them off in the hope of seeing our
collective name in print before too long. This illustrated
one of the main objectives of the weekend, namely engendering team spirit
and getting to know each other better.
We also had to attend to mundane practical matters. Christopher had
already issued his “Marching Orders” and the final details of our
itinerary were checked.
This gathering formed a very important part of our preparations. That it
took place at all and, in particular, in Birmingham was due to the hospitality
of Gwyneth
Gardiner whose normal household of
7 became a mini-hotel for 15. Fortunately,
the weather was fine and some of the younger Gardiners were able to sleep
under
canvas in the garden. Other countries may have had more money lavished on
their final preparations but none could have enjoyed such warm and generous
hospitality and such good food as we received. We
are all most grateful.
And so to IMO itself.
34th IMO: Some Statistics
A total of 413 contestants from 73 countries took part.
The UK team finished 14th out of 73 with 118 points (out of 252).
Each of the 6 team members won a medal, the haul being
3 Silver Medals and 3 Bronze Medals.
The top four teams were
China 215, Germany 189, Bulgaria 178, Russia 177.
A total of 118 questions were proposed by 43 participating countries.
All 3 UK proposals were short-listed, with one being chosen as question
2 (see below) and a second almost being chosen. The latter two were both
composed by David Monk (Edinburgh) and the other UK proposal was by
Christopher Bradley.
Question papers were prepared in 47 different languages.
The Questions
All contestants sit two papers on successive days. Each paper contains 3
questions, each question being worth 7 points.
On each day the time allowed was 4½ hours.
The questions were proposed by the countries indicated.
FIRST DAY
-
Let f(x) = xn + 5xn-1 + 3 where n
> 1 is an integer. Prove that f(x)
cannot be expressed as the product of two polynomials, each of
which has all its coefficients integers and degree at least 1.
(Ireland)
-
Let D be a point inside the acute-angled
triangle ABC such that

and
AC.BD = AD.BC.
- Calculate the value of the ratio
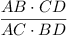 .
.
- Prove that the tangents at C to the
circumcircles of the triangles ACD and
BCD are perpendicular.
(United Kingdom)
-
On an infinite chessboard, a game is played as follows.
At the start, n2 pieces are arranged on the
chessboard in an n × n block of adjoining
squares, one piece in each square. A move in the game is a jump
in a horizontal or vertical direction over an adjacent occupied
square to an unoccupied square immediately beyond. The piece
which has been jumped over is then removed.
Find those values of n for which the game can
end with only one piece remaining on the board.
(Finland)
SECOND DAY
-
For three points P, Q,
R in the plane, we define m(PQR) to
be the minimum of the lengths of the altitudes of the triangle
PQR (where m(PQR) = 0 when
P, Q, R are
collinear).
Let A, B, C be
given points in the plane. Prove that, for any point
X in the plane,

(Macedonia)
-
Let N = {1, 2, 3, ...}.
Determine whether or not there exists a function
 such that
such that
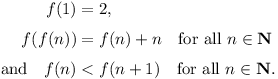
(Germany)
-
Let n > 1 be an integer. There are
n lamps L0, L1,
..., Ln-1 arranged in a circle. Each lamp is
either ON or OFF. A sequence of steps S0,
S1, ...,Si, ... is carried out.
Step Sj affects the state of Lj
only (leaving the state of all other lamps unaltered) as
follows:
if Lj-1 is ON,
Sj changes the state of Lj from ON
to OFF or from OFF to ON;
if Lj-1 is OFF,
Sj leaves the state of Lj
unchanged.
The lamps are labelled mod n, that is
L-1 = Ln-1 ,
L0 = Ln ,
L1 = Ln+1,
etc.
Initially all lamps are ON.
Show that
- there is a positive integer M(n) such that
after M(n) steps all the lamps are ON again;
- if n has the form 2k then all
the lamps are ON after n2 - 1 steps;
- if n has the form 2k + 1 then
all the lamps are ON after n2 - n + 1 steps.
(The Netherlands)
How the UK Team Performed
| Q1 | Q2 | Q3 | Q4 | Q5 | Q6 | Total | Medal |
|---|
| Tom Fisher | 1 | 1 | 7 | 5 | 7 | 7 | 28 | Silver |
| Alistair Flutter | 7 | 0 | 1 | 1 | 6 | 0 | 15 | Bronze |
| Catriona MacLean | 7 | 0 | 7 | 0 | 6 | 0 | 20 | Silver |
| Alex Paseau | 7 | 0 | 1 | 2 | 7 | 0 | 17 | Bronze |
| Luke Pebody | 1 | 4 | 6 | 4 | 7 | 5 | 27 | Silver |
| Chuan Tze Teo | 1 | 0 | 4 | 3 | 2 | 1 | 11 | Bronze |
| Total | 24 | 5 | 26 | 15 | 35 | 13 | 118 | |
|---|
It is tragic to see that our worst performance by far was in the geometry
question that the UK had proposed! Students (and teachers)
who wish to take Mathematics seriously must make greater efforts to
come to terms with basic geometry. (A series of four booklets to help
them do just this is available at a cost of £2 each from Tony
Gardiner at the above address; make cheques payable to “BMOC”.)
Overall Performance of All Contestants
When the questions were finally selected, many of us felt that we were
setting the contestants a very stiff challenge. This was confirmed by the
marks. Only 2 contestants (one each from China and Taiwan) scored the
maximum of 42. A contestant from Germany scored 39. The next best score
was 37. The range of scores for the various medals was as follows:
| Gold | From | 31 | to | 42 | (35 contestants) |
| Silver | From | 20 | to | 30 | (66 contestants) |
| Bronze | From | 11 | to | 19 | (97 contestants). |
Only 12 teams scored at least half marks (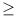 126). There was a big gap between
Canada and Israel who were 18th equal with 113 and Japan who were 20th
with 98. Some countries did not field a full team of six. (It is
worth mentioning that the IMO is meant to be a competition for
individuals not teams. However, more attention is usually paid to
team performances.)
126). There was a big gap between
Canada and Israel who were 18th equal with 113 and Japan who were 20th
with 98. Some countries did not field a full team of six. (It is
worth mentioning that the IMO is meant to be a competition for
individuals not teams. However, more attention is usually paid to
team performances.)
How the 34th IMO was run
The organisation was superb and no expense was spared in making sure that
everything went as planned. (It is perhaps not irrelevant to remark that
Istanbul is one of the cities involved in the final contest to host the
other Olympics in the year 2000.) Team Leaders, Deputy Leaders and Teams
all received some spending money (a few hundred thousand Turkish liras!),
an IMO T-shirt, badge, posters and a shoulder bag (for the Teams) or canvas
brief case (Leaders and Deputy Leaders).
A full
programme of excursions was arranged to enable us to make the best use of
what spare time we had.
(See the diary below.)
Transporting 800 people from A to B requires an operation of military
precision, particularly so when most of the 800 are mathematicians! Each
team was allocated a guide, usually a local student who could speak the
appropriate language. The guides made sure that the teams were at the right
place at the right time. Events elsewhere in Turkey meant that security
was an important matter. A security person travelled on every bus and
escorted us on excursions. (This also helped to make sure that there was no
contact between Team Leaders and their Teams/Deputy Leaders until after the
end of the second paper.) The cavalcade of 20 buses made an impressive
sight as it swept along with a police car at the head and an ambulance at the
rear. The latter was a sensible idea. If you take a large group of
adolescents anywhere, there is a sporting chance that someone may suffer cuts,
bruises or a sprained ankle. Fortunately, the ambulance did not seem
to be needed, although 10 contestants were sick after eating some
water-melon.
All decisions concerning the competition were in the hands of the Jury which
consisted of the 73 Team Leaders, each of whom had one vote. We were each
given a red plastic wand with our own 3-letter code marked on it. The UK
code was UNK so that I became “The Man From UNK”. Votes were frequent and
consisted of the chairman counting the number of raised wands.
Jury meetings were like a scaled down version of the
House of Commons. On occasions, the whole thing threatened to turn
into a bear garden as an argument broke out, usually in a foreign
language, among some of the more volatile Leaders.
The business of the Jury was conducted principally in
English but interpreters were on hand to provide translations into French
and Russian. (German did not seem to be required.) Our main tasks were to
select the problems to be set, approve the various translations, confirm the
marks scored, award medals and deal with any other matter related to the IMO
regulations. That we succeeded in achieving this was a great credit to the
person who chaired the majority of our meetings. Professor Semih Koray
earned the respect of everyone by conducting the business firmly but with
charm and tact.
The other major task for the Leaders and Deputy Leaders was the marking of the
scripts. We each marked the scripts of our own Team and then had to justify
our marking before a panel of Co-ordinators. A schedule was drawn up
whereby each country was allocated 6 half-hour slots over a 3-day period.
In each slot the marking of one specified question was discussed.
Inevitably, some difficulties arose and the schedule was knocked well off
course. Some teams were still going strong (?) at 3 o’clock in the
morning when the session should have finished at 22.30.
Fortunately Christopher Bradley and I were not affected by any major delays
and we felt that the Co-ordinators had been tough but fair. Here again I
should like to single out one person for special mention. Professor Albert
Erkip was responsible for co-ordinating the Co-ordinators as well as
chairing the group which short-listed the problems for the Jury. He also
superintended the production of the 47 translations and the posting of
marks on the display boards as the drama unfolded. All of these tasks he
discharged with remarkable sang-froid and good humour. For me, Professor
Koray and Professor Erkip were two people who played a huge part in making
the 34th IMO such a success.
To give a flavour of what really happened I offer a
Diary of The Man From UNK
Tuesday 13th
Arrive in Istanbul at 18.00. Taken to the hotel which is to be our
base for the next 5 days. Very posh, very American. After dinner,
first Jury meeting at 21.00 is brief and we all go off to tackle the
short-listed problems (without peeping at the solutions).
Wednesday 14th
In the hotel all day, with Jury sessions at regular intervals. As
time passes, the short list gets shorter and shorter.
Thursday 15th
We get down to 4 possible (non-disjoint!) sets of 6 questions and
these are eliminated one by one. In 2 of the 4, there are 2 questions
proposed by the UK but the final choice has just one UK question. I
find myself in charge of the group preparing the official English
versions of the questions. Those involving the chessboard and the
lamps could be tricky. Taken to an evening concert of music for
chamber choir. The choir scores highly for effort but a choral
arrangement of “Ich grolle nicht” from Schumann’s Dichterliebe
is less successful (to be rivalled only by the UK team’s performance
on Question 2!). Work until 02.00 to get the lamp problem licked into
shape.
Friday 16th
Official versions of the problems in English, French, German, Russian
and Spanish approved before lunch. Rest of the day highly frustrating
as Leaders prepare the other 42 translations. Modern technology is
wonderful if it works but the software fails to cope. Many Leaders
give up and write things out by hand. Many errors to be eradicated.
Final approval of all versions only possible after midnight.
Meanwhile Christopher and the Team will have arrived, I hope!
Saturday 17th
Opening Ceremony at 10.00. Leaders arrive at 09.00 and are shepherded
into the gallery. Teams and Deputy Leaders arrive a little later and
are put in the stalls. Leaders know the questions; so we are held
incommunicado. Spot Christopher and the team with distinctive
T-shirts (courtesy of Luke and family). My T-shirt is delivered by
air mail (thrown up to the gallery from below). Ceremony starts with
a curious modern ballet. Learn later that it had something to do
with telephone chatlines. A few speeches including one by the Deputy
Prime Minister which I fail to understand because someone has pinched
my headphones (for simultaneous translation). Best speech is by a
young university student who competed in IMO in 1991. Leaders taken
off on excursion until dinner. Visit the “Blue Mosque”, Aya Sofya
and Topkapi Palace. Get first real glimpse of the Bosphorus from
Topkapi. Scenery stunning. Can’t wait for tomorrow’s boat trip.
Sunday 18th
First paper from 09.00 to 13.30. During first half-hour, contestants
may send written queries to the Jury if they need any clarification
about what a question means. Queries are brought to the Jury by
runner from the exam hall next door and are answered in order of
receipt. Some get a dusty response such as “No comment” or “Read
the question” but others are treated more sympathetically where it is
felt appropriate. Only 30 questions received, rather fewer than
usual. The efforts expended on preparing the official versions have
not been in vain. Leaders leave to take part in the “Parade of
Boats” marking the end of a festival of history and the sea. Five
little puffers have been decorated with IMO banners. Sail up towards
the Black Sea and then back down in procession. Glorious afternoon,
nice breeze to temper the heat, magnificent scenery. Back to base to
collect scripts from the morning exam. Stay up until 1 a.m. marking.
Disaster on question 2!
Monday 19th
Leaders moving to join Deputy Leaders after second paper. Teams will
be close by. Jury receives 52 queries. Then bad news! During the
first paper, one contestant was found to have a pocket book of
formulae in the exam hall. Subgroup set up to ascertain the facts.
Full Jury meeting called for 22.30.
Meet team at end of exam. They feel that they have done quite well on
the second day, especially in question 5, but are apprehensive about
the first day. Meet up with Christopher who has been on an excursion.
Examine co-ordination schedule. Foresee a very long night ahead.
Scripts for second paper arrive around 18.30. We must have the lamp
question sorted out completely by 09.00 the next morning.
First co-ordination at 21.00. This is the chessboard question and
goes well. (We did a lot of chessboard problems during training,
though none quite like this.) Then at 22.00, Christopher and I change
sides and become co-ordinators of the Turkish responses to our UK
geometry question. Running behind schedule now and I miss start of
Jury meeting. Contestant deemed to have cheated and is disqualified.
Worse is to follow! On the second day two contestants were found in
possession of calculators in the exam hall, contrary to the rules.
Another subgroup set up to investigate. Back to room at midnight to
deal with the lamp question. Spend 3 hours with Christopher trying to
unscramble Luke’s solution with partial success. Bed at 04.00.
Tuesday 20th
Four co-ordinations throughout the day. We get 5 out of 7 for Luke’s
lamps. Burning the midnight oil was worth it. Marks start to appear
on the display boards. By 22.30 we have a fair idea of where we shall
finish, somewhere between 12th and 17th. The worst of our labours
seem to be over.
Wednesday 21st
Last co-ordination over by 09.30. Spend morning watching final pieces
of jig-saw appearing on display boards. Spend afternoon in downtown
Istanbul, including a visit to the Covered Bazaar. Run the gauntlet
of people trying to sell us perfume, jewellery, leather jackets,
carpets and much more.
Final Jury meeting starts at 21.00. Two contestants with calculators
are awarded 0 points for the second day. Marks for all students
approved and distribution of medals agreed. Then trouble!
Allegations that one question had been used in an earlier competition
and could have been familiar to some contestants. Chairman calls a
short break to allow things to calm down. Some contestants have
arrived to find out the results. Meeting resumes at 23.30. Report
from Site Committee (in future to be called Advisory Board) which
keeps things on the move between IMO’s. Host countries for future
years are noted and approved (up to 1998). Discussion on method of
selecting problems and possible changes to regulations. A few more
votes and we end at 01.10.
Thursday 22nd
Quiet morning. Send a FAX to Tony containing the main statistics.
Then we are all at sea. About 800 people pile aboard a huge ferry for
an 8-hour cruise on the Sea of Marmara and the Bosphorus. We visit
the Princes’ Islands and then stop to take on provisions. Now it
really is party time. A disco is in full swing on the middle deck
with the young (and some of the not so young) from all over the world
dancing and singing. I spend most of the time at the stern watching
this amazing sight while soaking up a glorious sunset. The scenery we
saw on Sunday in bright sunshine is just as beautiful in the dusk.
Bus journey back very quiet. Most people have crashed out.
Friday 23rd
The team, Christopher, John Hersee (Secretary of the Site Committee)
and myself pay an early morning courtesy call to the British Council
and meet the Consul (Cultural) Istanbul who has taken a keen interest
in our visit. Hail 3 taxis to get us back to the hotel. The 3
drivers seem to be pals and decide to have a race. All driving in
Istanbul is crazy but this taxi journey is horrendous! Closing
Ceremony starts at 14.30 with some enjoyable singing and dancing.
Then comes the prize-giving. Specially loud cheers for Turkish
medal-winners and a girl from Bosnia. The Croatians prove to be a
lively bunch and Katy’s hat takes a trick. Various speeches follow
with everybody congratulating everybody else. It is Professor Koray
who epitomises the whole spirit of the occasion by reminding us that
MATHEMATICS IS A UNIVERSAL LANGUAGE
uniting all countries of the world. Then as the lights dim, all the
guides and other helpers march in with luminous sticks. Everyone
sings “We are the world, we are the children” and we all vow to meet
again in Hong Kong in 1994. It would be a strange person indeed who
could fail to find this occasion intensely emotional.
Time to relax and celebrate informally. We cross the Bosphorus and I
set foot in Asia for the first time (and have a certificate to prove
it). We go to a forest which forms the highest part of Istanbul.
Party time again! Take a picture of our team together with
representatives of Canada, Colombia, Croatia, Ireland, New Zealand and
Slovakia. Discover a Hungarian who has already managed to lose his
gold medal! A wedding party appears. I bet they got a big surprise
to find not teddy bears having a picnic but 800 mathematicians. All
the while young entrepreneurs aged about 5 invite us to buy postcards
and chewing gum.
Finally the cavalcade makes the short trip to Beylerbeyi Palace for a
banquet. No formal speeches, just everyone dining alfresco under the
magnolias beside the Bosphorus with an orchestra playing. By this
time Alistair has lost and recovered just about everything and the
Hungarian has been reunited with his gold medal. So everyone is
happy.
Saturday 24th
Team roused at 06.10 by their guide, the indefatigable Alp whose great
efficiency and cheerfulness were much appreciated by all of us,
especially by Christopher. Everybody gets to the airport safely.
Most of the team sleep on the flight home. Katy finds the room key
lost several days earlier. Arrive on time at Heathrow to be met by
parents.
Concluding Remarks
The 34th IMO was an unforgettable experience for all those lucky enough to
be there. Not everyone was a winner but at the disco or in the forest
nobody was worried. Young people from all over the world (including some
of its most troubled regions) were enjoying themselves, exchanging addresses,
signing T-shirts and making friendships which may last throughout their
lives. The universal language of Mathematics was the catalyst that brought
this about. It was a great honour and enormous pleasure to lead the UK
team and I congratulate them on their achievement.
Our thoughts now turn to the 35th IMO to be held in Hong Kong during the
period 8th-20th July 1994. Soon the process of picking the UK team will begin.
Along the way there will be winners and losers. However, we should
do all we can to enable as many talented youngsters as possible to
experience the unique character of this
universal language of Mathematics.
I encourage all of you to read again my
initial remarks about Mathematical Competitions. Since we now seem
to be back where we started, it is time to stop.
Acknowledgements
My personal thanks go to Christopher Bradley and Tony Gardiner for their
help and support which has been so generously and freely given. Tony’s
myriad contributions to IMO, BMOC and related activities deserve the
highest praise. BMOC is grateful to its participating societies, namely,
the Edinburgh Mathematical Society, the Institute of Mathematics and its
Applications, the London Mathematical Society and the Mathematical Association.
BMOC is deeply indebted to its sponsors, including the Department for
Education and the Royal Society. Special thanks are due to Trinity College,
Cambridge for its support, particularly for hosting the Training Session
in April.
Return to IMO Register home page
Contact: Joseph Myers
(imo-register@imo-register.org.uk)
Online HTML version last updated:
5 January 2016
![]()
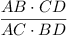 .
.