Balkan Mathematical Olympiad 2015, Leader’s report
L. Alexander Betts
3rd–8th May 2015
Summary, observations and thanks
This is the UK/ROI team leader’s report on the 32nd Balkan
Mathematical Olympiad, held in May 2015 in Athens. Our team comprised
five British students (Alex Harris, Lawrence Hollom, Sam Kittle,
Kirsten Land and Philip Peters) and one Irish student (Luke
Gardiner). The team was accompanied by three adult volunteers: myself
(leader); Gerry Leversha (deputy leader); and Jill Parker
(pastoral). The competition consisted of a single four-and-a-half hour
exam paper comprising four questions, one from each of the standard
olympiad areas of algebra, geometry, combinatorics and number
theory. The questions were as follows.
Let a, b and c be
positive real numbers. Prove that
a3b6 + b3c6 + c3a6 + 3a3b3c3
≥ abc(a3b3 + b3c3 + c3a3) + a2b2c2(a3 + b3 + c3)
Let ABC be a scalene triangle with incentre
I and circumcircle (ω). The lines
AI, BI, CI intersect
(ω) for the second time at the points
D, E, F,
respectively. The lines through I parallel to the sides
BC, AC, AB intersect the
lines EF, DF, DE at the
points K, L, M,
respectively. Prove that K, L,
M are collinear.
A jury of 3366 film critics are judging the
Oscars. Each critic makes a single vote for his favourite actor, and a
single vote for his favourite actress. It turns out that for every
integer
n ∈ { 1, 2, …, 100 }
there is an actor or actress who has been voted for exactly
n times. Show that there are two critics who voted for
the same actor and for the same actress.
Prove that among any 20 consecutive positive
integers there exists an integer d such that for each
positive integer n we have the inequality
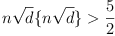
where {x} denotes the fractional part of the real
number x. The fractional part of a real number
x is x minus the greatest integer less
than or equal to x.
The students performed well, especially given that for half of the
students this was their first international competition. All in all,
we obtained four bronze medals, mainly due to a solid performance on
the first two questions. The precise breakdown follows.
| Q1 | Q2 | Q3 | Q4 | Total | Result |
|---|
| Luke Gardiner | 3 | 10 | – | – | 13 | Bronze |
| Alex Harris | 10 | 10 | 0 | 0 | 20 | Bronze |
| Lawrence Hollom | 10 | 1 | 0 | 1 | 12 | Bronze |
| Sam Kittle | 0 | – | 2 | 0 | 2 | – |
| Kirsten Land | 0 | 1 | 0 | – | 1 | – |
| Philip Peters | 10 | 10 | 0 | 0 | 20 | Bronze |
We are greatly indebted to everyone who made the Balkan Olympiad a
great success this year. Our particular thanks go to the chair of the
jury, Prof. Theodoros Bolis, and the chair of the problem selection
committee, Prof. Anargiros Fellouris, along with all of the other
organisers and guides who contributed to the smooth running of the
event. Of course, we are also grateful to everyone on the British and
Irish side who helped train the students and foster their enthusiasm
for mathematics. These volunteers and family members are too numerous
to mention by name, but we would like to single out Bev Detoeuf of the
UKMT with all her help with the logistics for the competition. Many
thanks to you all.
Leader’s diary
Disclaimer: The leader’s diary has two main purposes:
to give everyone an insight into what goes on behind the scenes in
international maths competitions; and in some small part to amuse. In
the cause of this, undue attention may have been placed on the various
mishaps that occurred – it should be understood that I found the
competition in general very pleasant, and don’t mean to imply
otherwise.
May 3rd
As planned, we meet at Heathrow Terminal 2 (“The
Queen’s Terminal”) at 10:30, and the students say goodbye
to their parents before receiving their ever-important UKMT
shirts. Having checked in online, we are directed to the self check-in
desks, where we obtain duplicate boarding passes, and thence proceed
to security.
We have left plenty of time to resolve any problems that come up
before our flight, and are not disappointed. Luke has not been allowed
to take a pair of compasses on his ultra-high security flight from
Ireland, and the airport staff aren’t impressed with
“Gerald” Leversha’s attempts to impersonate the
“Gerry” Leversha that has been booked in. Additionally,
there has been a mix-up with the registration fees, and Gerry has to
step in temporarily, withdrawing enough cash to prop up the Greek
economy during our trip. Despite the delays, we still clear security
in good time and board our flight to Athens.
After an uneventful flight, we arrive in Athens airport and I say
farewell to the students as I am whisked off to the leaders’
hotel for the business of selecting the papers. I am still a little
early for dinner, so I hang around watching the sea and chatting to
the chair of the jury, Theodoros Bolis, who is a very amiable
chap. After dinner, we proceed to the jury room, complete with disco
ball, and are presented with copies of the problems. These are
generally of a very high standard, and I stay awake late into the
morning trying to solve a particularly beautiful combinatorics
problem.
Deputy leader and observer1
Once I’ve been separated from the students, they are taken
with Gerry and Jill to the seaside hotel where they will be staying
for the duration of the competition. There, the students are
introduced to their guide, who seems quite taken aback that the team
would rather read and study than swim in the sea the other side of the
road.
While the students are in their guide’s hands, Gerry and Jill
are informed that there is no room in the hotel for them, and they
have been put in a separate hotel, which according to Gerry is located
roughly at the edge of the observable universe. This is particularly
unfortunate for Jill, who is here in a specifically pastoral
capacity. Still, there is little they can do in the immediate future,
so they take a taxi to their accommodation.
1For the first few days I was
not allowed to contact the students, deputies, observers or the
outside world in order to protect the integrity of the shortlist. Thus
my account of their first few days is largely patched together from
what they would tell me later in the competition.
Star Wars Day
Regretting my late night shortlist party, I wake up begrudgingly
and stand under the shower. This has only a single control, which
seems to affect flow rate and temperature simultaneously – woe
betide the man who likes his showers cold and pummelling. Fortunately
I am not he, so I head to breakfast refreshed, where I meet Massimo
and Vesna, the Italian and Macedonian leaders.
The first task for the day is to receive the official solutions and
continue working on the problems. However, before long we are herded
into a coach to join the students for the opening ceremony, which
takes place at their hotel. The podium is set up like a mini UN, with
a long table for the leaders covered with small national flags. I find
my seat next to the Irish flag, and my other seat next to the union
flag, so I perform a quick and politically unlikely amalgamation and
sit down.
The ceremony is very pleasant. One of the organisers clearly
understands the virtue of brevity, and the whole thing runs like
tightly-wound clockwork. After a few short speeches, we proceed to the
parade of teams, which this time just consists of the teams standing
up when their name is called, like some sort of international
Whack-a-Mole. All the students seem very pleased to be here (as they
should be), and the Turkmen and Azeri teams are showing off some
snazzy uniforms. Not that the UK/ROI team notice, the peculiar L-shape
of the room has rendered half the students invisible to the other
half.
Still, it was an enjoyable ceremony, and I give a wave and smile to
the team as I return to the leaders’ hotel for the main event:
the deciding of the paper. We begin by weeding out the known problems,
and categorise the remainder in terms of difficulty. Suddenly problem
1 is selected: an easy-ish inequality that sadly rules out many
beautiful abstract algebra problems. Massimo and I exchange looks. In
a second snap poll, the jury takes up problem C1, at the expense of a
very challenging combinatorics problem I had had my eye on. Gruntled I
am not.
However, at this point the jury realises it’s about to select
the paper too quickly, so we entertain a long debate on how to decide
the remaining questions. Eventually, N6 (a Serbian proposal, and for
me the question on the shortlist) becomes our hard problem. The
Romanian leader invokes the spirits of Thales and Euclid in support of
the purest geometry questions on the shortlist, and accordingly the
jury takes G4 to complete the paper.
Then Fawzi, the Saudi leader, and I get to work producing the
official English paper. We completely rephrase the combinatorics
question, and I take the opportunity to expunge the non-words
“favorite” and “center” from the paper. The
jury accept our wordings, although while I’m out of the room,
the 3366 film critics in problem 3 mysteriously all become male. It
seems that we will be finished with the work well in advance of
bedtime.
However, it is not to be so. After dinner, I detect a flaw in
problem G4, and decide to hit the jury with the nuclear option:
problem 2 on our paper is just a degenerate case of Desargues’
theorem. Normally, this would rule it out as a suitable problem, but
this time my nuke falls on deaf ears. After this, it doesn’t
take long to resolve the matter of the remaining mark schemes, and I
head to bed. Security forbids me from wishing the students luck, but I
have my fingers crossed for them.
Deputy leader and observer
Gerry and Jill spend their day looking for hotels closer to the
students, but to no avail. Nonetheless, the beneficent hand of Fate
rewards their efforts when the organisers suddenly find a place for
Jill in the students’ hotel, and she is promptly carted off to
her new lodgings. No corresponding room appears for Gerry, however,
and for the remainder of the competition he remains based in his hotel
somewhere in the vicinity of Betelgeuse. Having to visit the
leaders’ and the students’ hotels regularly, he quickly
becomes a regular patron of the Athenian taxi service, from which he
learns much about the Premiership efforts of Manchester United.
May 5th
Today is contest day. After some collective dawdling, the leaders
board their bus to meet the students at the contest site, the
mathematics department of the University of Athens. This boasts
stunning views over the surrounding hillside, although we don’t
have much time to appreciate this before we head into the building. We
are sitting in a room nearby the contest hall, and the students have
the opportunity in the first half hour to ask written questions of
clarification, which we are there to answer. One of the Serbian
students puts forward the question “Does problem 1 have to be an
inequality again?”, but the remaining questions are
disappointingly sensible.
After answering the questions, we still have four hours to kill
before the students finish the exam, so we head to the Acropolis
museum for a guided tour. With the aid of several models, our guide
explains the history of the Parthenon as a place of worship variously
Hellenic, Christian and Islamic. We then continue into the main body
of the museum, where I find the remaining paintwork on the ancient
statues particularly interesting.
After lunch, I head to the students’ hotel to chat with them
after the exam. However, the exam started an hour late and their bus
takes an age to return, so we only rendez-vous shortly before four. I
say a brief hello before diving out of the stampede for the buffet,
worried that in the seven hours since breakfast, the students may have
turned feral. Over lunch, we have a more civilised chat. It seems a
good proportion of our students are claiming solutions to either
problem 1 or 2, but progress on problems 3 and 4 is thin on the
ground.
Later, I retire to the leaders’ hotel to mark the
scripts. The scripts are due to arrive at 6pm, but they aren’t
anywhere to be seen. I take the opportunity to send a lot of emails,
but I have run out of things to send long before the scripts finally
limp through the door at 10 o’clock. Despite the very late hour,
I summon Gerry from his hotel in the Oort cloud and we have a first
look through the scripts.
The solutions claimed by the students seem pretty solid generally,
though Lawrence’s partial trigonometric solution to question 2
seems unsavable. As they predicted, Sam and Kirsten don’t have
much, but Sam’s work on problem 3 looks tantalisingly close to a
new solution. I stay up trying to find a quick fix to Sam’s
crucial lemma, and by 3am I succeed and collapse into bed.
May 6th
Today, the students and Jill are out visiting the Acropolis museum
and exploring, giving them some well-earned relaxation. Gerry and I
are up for coordination, which entails meeting with some local
organisers and agreeing the marks with them. The coordinators have
been provided with photocopies of the students’ scripts, but not
all of the scrap paper has been photocopied, so occasionally we need
to provide this.
Our first coordination is question 3, with a pair of Greek
coordinators playing the classic Good Cop/Bad Cop routine. We agree to
five non-contentious zeroes, and then begin examining Sam’s
script. I explain to the coordinators how to fix Sam’s crucial
claim, and run them through how he concludes. The coordinators seem
surprised that this approach works, but happy that this is worth
non-trivial marks. I suggest three, but Bad Cop points out that Sam
has made a minor error in his introduction, so in the end we agree
that 2 is the fair mark. The whole thing is very civil, and I am in a
good mood to face the other coordinations.
Problem 4 is up next. I don’t think we have anything worth
any marks, except perhaps a little comment in Lawrence’s rough
work, which identifies the crucial bounding that needs to be done to
connect to the number theory. The coordinators feel this is worth a
mark, and we are inclined to agree.
Surprised and happy, we head next to the problem 1 coordination,
with two very cheerful coordinators. Three tens and two zeroes are
quickly agreed, and then we turn to Luke’s script. The
complicating factor here is that, due to running out of time in the
exam, Luke has concludes his proof appealing to Schur’s
inequality, which his instincts have rightly told him is the final
crucial step. However, he told us that he didn’t have time to
work out the details of the Schur argument, which are quite subtle, so
we argue that his script is an especially meritorious attempt. Pleased
with our honesty, the coordinators agree, and after a short discussion
we settle on three marks.
The final coordination occurs quite late in the day, and the
problem 2 coordinators, no doubt keen to get home, seem in a generous
mood. The tens and the zeroes are quickly settled, and Kirsten picks
up a single point for a useful remark in her rough work. In
Lawrence’s incomplete trig bash, he has also made a useful
non-trigonometric observation, and he too is rewarded with a
point.
Once the coordinations are finished, we have the final jury
meeting, where the chair of the jury passes on to the organisers of
the next Balkan Olympiad. If all goes to plan, the next hosts should
be Albania, but the Macedonians are ready to step in should they have
difficulties. In any case, the competition should be in safe hands for
the next year, and I wish them the best of luck in organising a
competition at least as enjoyable as this year’s.
The main event of the meeting, though, is the setting of the medal
boundaries. As expected, 20 marks isn’t enough for a silver
medal, but the bronze boundary is unusually low at 12 marks, meaning
that four of our students get bronze medals. This is great news, and I
find the team to let them know. They are suitably pleased, and the
rest of the day passes in an enjoyable and exhausted blur before I
head back to my hotel to crash out.
May 7th
Today is the day of the closing ceremony and presentation of
medals. We expect that the UK media are full of reports on the
competition, but we hope that more minor news stories are not totally
eclipsed.
Before the ceremony, we all board buses for the group excursion to
the site of the battle of Marathon, taking a scenic route over a
mountain pass. The site itself isn’t particularly
impressive. Aside from some informative signs near the entrance and a
central tumulus, there is not much to see except fields. I content
myself with photographing interesting flowers and blue
butterflies.
After our short visit, we head to the beach for a break. Gerry,
Jill, Kirsten, Sam and Luke head off to get a coffee, while Alex,
Philip and I paddle in the shallows. The water is beautifully cool and
clear, and we can see tiny fish scurrying off into the seaweed. We
spot some tiny crabs, and have to dissuade Philip from trapping them
in his water bottle. Somehow I can’t see us getting that through
customs.
After an iced chocolate, we head back to Athens for the closing
ceremony and dinner. The festivities begin with a series of addresses
from various mathematical dignitaries, beginning in English, but soon
switching into Greek. One speaker talks (in Greek) about how maths is
a universally understandable language, while another (also in Greek,
but with translations provided) talks about maths being a pylon of our
civilisation. After the initial addresses, we are treated to a few
piano pieces from the next Vangelis, and a fascinating display of
Greek folk dancing which gets one of the local organisers leaping up
and down the aisles.
After this is the presentation of the medals. There is one perfect
score from a Turkish student, and only six gold medals in total, all
of which are very well-deserved in the face of this tough paper. There
is much cheering for the home team, but Gerry, Jill and I put in a
good effort as our four medallists drape themselves in their
respective flags and are given their medals.
After the presentation is over, we walk back to the hotel for a
final dinner together, and the students gradually disappear to get
some sleep for the flight back the following day.
May 8th
Despite an obscenely early start, the return journey to the UK
passes without incident, and the British students are reunited with
their parents at the terminal. We say our goodbyes, and to Luke as he
boards his flight to Ireland, and the Balkan Olympiad is over for
another year. We very much enjoyed the competition, and am sure will
all have fond memories.
Return to IMO Register home page
Contact: Joseph Myers
(imo-register@imo-register.org.uk)
Online HTML version last updated:
21 April 2016
![]()